Foundations of Math: Teaching Students with Significant Disabilities

With the right supports and knowledge, all teachers and learners can engage in the teaching and learning of mathematics. This course includes all components of Foundations of Math with the addition of research and classroom video specific to learners with significant disabilities and complex communication needs.
Educators in self-contained and center-based environments will experience approaches to assessment and instruction that will help learners develop meaningful mathematical understanding.
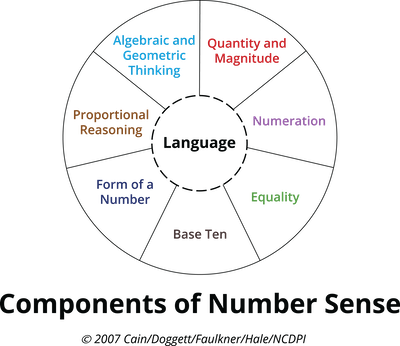
What are the Components of Number Sense?
Reading is not reading if all of the components of reading are not working together (such as fluency, comprehension, vocabulary, phonemic awareness, and phonics). Math is not math if learners are not engaging in, and making connections between, multiple components of number sense.
The phrase “Number Sense” is commonly used, and means different things to different people.
In this training, number sense refers to the integration of the 8 components presented in the Components of Number Sense “wheel,” each component connected to every other component through language. During the training, each component is defined, and participants learn classroom applications, diagnosis questions, and research related to the component.
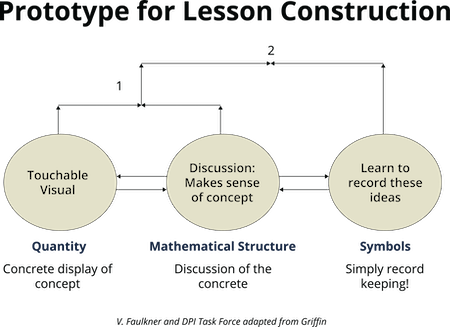
What is the Prototype for Lesson Construction?
Math in the course is taught using the Prototype for Lesson Construction. Participants see it modeled, work through it themselves, and have time to adapt their own lessons to fit this framework.
The prototype is based in developmental and cognitive research about how the brain learns math.
Arrows in the diagram show the need to first build relationships between quantities and language. Second, learners extend that knowledge to understand relationships between quantities, vocabulary, and discourse. Two way arrows between the ovals indicate the need to be fluent between representations, and move among and between quantity, structure, language, and symbols.
Why Try Something New?
Learners can and should receive a cohesive and coherent mathematics education. That education should start with gaining a solid foundation of math understanding so that they can make sense of their world, interact in it, and contribute to it.
Educators who understand this, but struggle to find curricular resources that help them provide this type of math education, may seek alternatives to what is available.
When current instructional approaches rely on disjointed topics or materials, memorization over understanding, steps to getting right answers, or repetitive tasks that do not deepen math knowledge, educators are right to seek an alternative.
This course is designed to deepen educators’ content knowledge and connect procedural knowledge to conceptual understanding. An emphasis is placed on constructing lessons that develop strong mathematical foundations in learners.
Evidence Base
- Mathematics in the 21st Century: What Mathematical Knowledge is Needed for Teaching Mathematics (Remarks made by Deborah L. Ball at the Secretary's Summit on Mathematics at the U.S. Department of Education in 2003.)
- Teaching Number Sense
- The Learning Trajectories
- Mathematics for Children with Severe and Profound Learning Difficulties by Les Staves
A Foundations of Math course was also developed for teachers who work in inclusive settings with students who study the Common Core State Standards. The pedagogy and philosophy are identical to Foundations of Math: Teaching Students with Significant Disabilities. However, this course does not include research, examples, and video of students with significant disabilities. Instead, it allows for planning and discussion related to students with disabilities who receive math instruction in general education classrooms or resource rooms and who are, or likely to be, working toward a high school diploma.
If you are interested in learning more, check out our full Foundations of Math video series on our YouTube channel.
